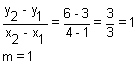Slope-Intercept Form
Sometimes, you have two points, but no graph. You can still find the equation of the line in slope-intercept form.
|
Step 1: Find the slope of the line. Step 2: Plug the slope of the line and either of the points into the general equation. Step 3: Solve the equation for b. Step 4: Write the equation. |
Example 1
Determine the equation of the line between (1, 3) and (4, 6) in slope-intercept form.
Step 1: Find the slope of the line.
Step 2: Plug the slope of the line and either of the points into the general equation.
y = mx + b
6 = 1(4) + b
Step 3: Solve the equation for b.
6 = 1(4) + b
6 = 4 + b
2 = b
Step 4: Write the equation.
We now know that m = 1 and b =2. Plug these values into the general equation.
y = mx + b
y = 1x + 2
So, y = 1x + 2 is the equation of the line between (1, 3) and (4, 6)