Solving Compound Inequalities (continued)

Now let’s look at a compound inequality with an “or” statement.

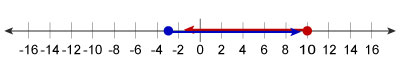
Solve and graph x – 8 ≤ 2 or 6x ≥ -18.

The first step is again to solve both inequalities.

x – 8 ≤ 2
x ≤ 10

6x ≥ -18
x ≥ -3

Now graph your solution.

Solving Compound OR Inequalities

This differs from our previous example because it is an "or" statement. That means that our solution could be located in either place. In this case, the two inequalities will overlap and cover the whole number line. The solution of this compound inequality is All Real Numbers.