Solving Compound Inequalities

Compound inequalities include the word and or the word or. You need to find the values of the variables that satisfy both inequalities. An example of a compound inequality is
x – 10 ≥ 0 and -5 + x < -6. You will also find problems that include an "or" statement. When solving these types of problems it is vital that you always graph the solution to see if it makes sense. There are times when your answer will be All Real Numbers or there may not be a solution. The graph will help you determine the validity of your answer.

Let’s take a look at some examples.

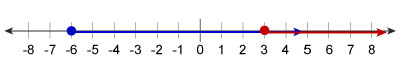
Solve and graph 5x ≥ 15 and x + 8 ≥ 2.

The first step is to solve both inequalities.

5x ≥ 15

x ≥ 3

x + 8 ≥ 2

x ≥ -6

Now graph your solution.

Solving Compound AND Inequalities

Notice that the inequalities both face the same direction. Since we are looking at an "and" statement, we want to know where both equalities are true. This happens at x ≥ 3. The final graph is

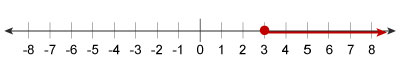
Solving Compound AND Inequalities