Conic Sections: Circles

Standard Equation of a Circle

Suppose you have the following circle centered at the origin.

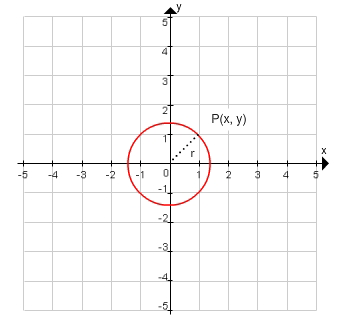
Circle with point P with coordinates x and y on the circle, the radius r from the origin to point P.

We can use the Distance Formula to find the length of the radius.

OP = r

square root of the quantity x minus 0 squared plus y minus 0 squared equals r, square root of the quantity x squared plus y squared equals r, x squared plus y squared equals r squared

This is the standard equation of a circle with a center at (0, 0) and a radius or r.