Surface Area
Before we begin to look at formulas and methods to find surface area, let’s discuss what surface area is. What does it mean when you are asked to find the surface area of a figure?
Let’s start with a simple box. This box is called a rectangular prism. A prism is a three-dimensional figure with two bases that are the same size and shape and are parallel to each other.
Take a look at the box below.
 |
Rectangular box |
If you wanted to cover this box with wrapping paper, you would need to cover all six sides. The area of all these sides added together is the surface area.
|
|
An efficient way to see what is needed is to imagine cutting along the edges and opening it up to lay it flat. This is often referred to as a ‘net’. Here is what this box would look like.
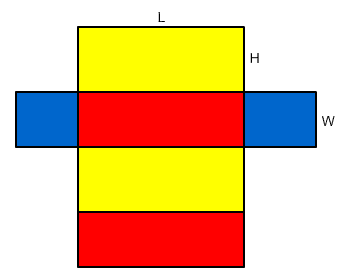 |
Sides of the box laid out flat |
Notice that there are six sides, two yellow sides (front and back), two red sides (top and bottom) and two blue sides (right and left).
If you can draw the surfaces of a three-dimensional object accurately, you will be able to calculate surface area.