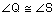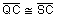Quadrilaterals and Polygons: Trapezoids and Kites

Properties of Kites

The last type of quadrilateral we are going to study in this unit is kites. 

Kites don't have any pairs of parallel sides. Instead, they have two pairs of adjacent, congruent sides.

 

Take a look at this kite.

kite with two pairs of adjacent sides marked congruent

 

Notice that the two adjacent sides meeting at the top vertex are congruent and the two adjacent sides meeting at the bottom vertex are congruent.

 

Properties of kites include:

  • the angles between non-congruent sides are congruent
  • the diagonals are perpendicular
  • the diagonal between the congruent angles is bisected by the other diagonal

 

Angles Between Non-Congruent Sides
Notice in the following image that the two adjacent sides to the left are congruent and the two adjacent sides to the right are congruent.

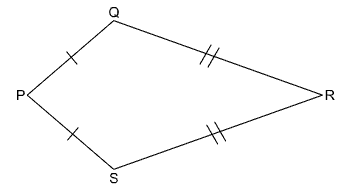
kite PQRS with sides PQ and PS marked congruent and sides QR and SR marked congruent

Angle Q and angle S are the angles between the non-congruent sides.

angle Q is congruent to angle S

Diagonals in a Kite
Take a look at the diagonals drawn in the kite below.

 

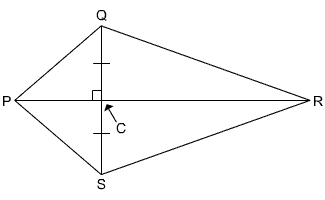
Kite PQRS with diagonals intersecting at a right angle at point C. segment QC and SC are congruent

 

Diagonals PR and QS are perpendicular.  You can see that they create four right triangles.

Triangle PQC is a right triangle.
Triangle RQC is a right triangle.
Triangle RSC is a right triangle.
Triangle PSC is a right triangle.

Also, segment QS, the diagonal between the congruent angles, Q and S, is bisected.

line segment QC is congruent to line segment SC