Domain and Range of Exponential Functions

The next step in really understanding exponential functions is to look at the domain and range of these functions.

The first thing you might notice is that there are no x-values which are excluded from an exponential function. No matter the "a", "c", "h", and "k" values of the function, any x-value can be used in an exponential function. This means that the domain of all exponential functions is All Real Numbers. Look at the graphs that you have created thus far in this section. Check any x-value and you will see that this domain is true.

The range of an exponential function depends on the vertical shift of the function. For example, look at the function f(x) = 2^x.

The asymptote of the function is at y = 0. This means that the function is defined at all values of y greater than zero. The range of this function is y > 0.

Now look at the function f(x) = 2^x + 2.

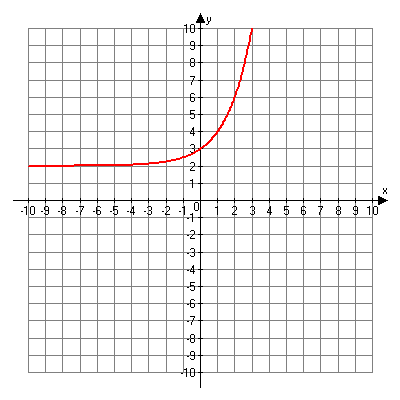
Now the asymptote is at y = 2 so the range of the function is y > 2.

It is important to remember the graph of an exponential function when asked to find the range, especially if a function is reflected. For example if the function f(x) = 2^x + 2 becomes f(x) = -2^x + 2, the range would become y < 2.

Next: Section B, page 9

© 2009 KC Distance Learning. All rights reserved.