Domain and Range and Logarithmic Functions
Let’s analyze the domain and range of logarithmic functions.

f(x) = log(x)
The logarithmic function is the inverse of the exponential function. If we look at the graph we notice that any y-value will work in the function. This means the range is equal to All Real Numbers. Only x-values greater than zero will work in this function since x = 0 is an asymptote. This means the domain is x > 0.
Let’s see what happens when the functions is transformed.
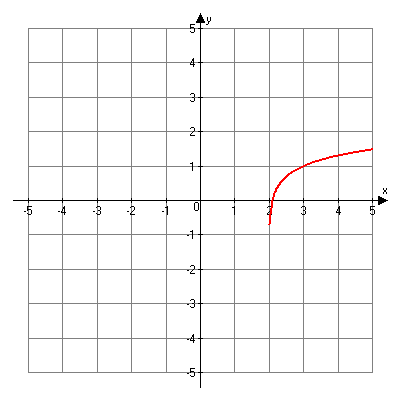
f(x) = log(x – 2) + 1