Logarithmic Functions

Logarithmic functions are oftentimes used to solve equations with variables in the exponents. The following is how exponential and logarithmic functions are related:

Click the play button ( ) below to listen to more information about logarithmic functions. Be sure to set your volume at a reasonable level before you begin.

Download a script of the audio.

The logarithmic function y = log_bx is the inverse of the exponential function y = b^x, where b ≠ 1 and b > 0.

The logarithmic function can be written in exponential form by using this conversion rule:

y = log_bx b^y = x

Logarithmic and Exponential Functions

The following is a list of logarithmic functions and their corresponding exponential functions:

y = log₃x 3^y = x

y = log₁₀1000 10^y = 1000

y = log₄x 4^y = x

y = log_ex e^y = x

Common Log

In the equation y = log_bx, if the b (base) is not written, the assumption is that the base is equal to 10. In other words log₁₀x = logx. This is called the common base or common log.

y = logx 10^y = x

The following is the graph of y = logx.

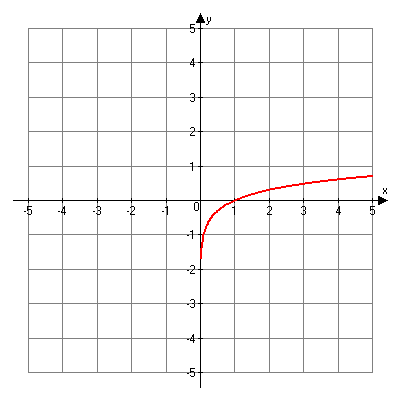
Graph of y is equal to log of x.
y = logx

Notice the asymptote of the logarithmic function is the y-axis or x = 0.

Natural Log

In the equation y = log_bx, the b (base) is sometimes equal to 'e'. The letter 'e' represents a special value:

e ≈ 2.71828

This value is seen often in science and business applications and will be studied more in depth in future math courses. This is called the natural log and is written:

log_ex = ln x

So...

y = ln x → e^y = x

The following is the graph of y = ln x.

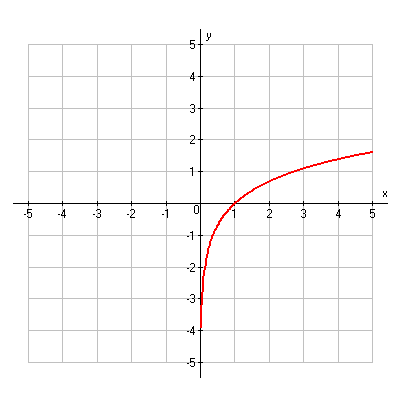
Graph of y is equal to natural log of x.
y = ln x

Notice the asymptote of the logarithmic function is the y-axis or x = 0.