Circumference of a Circle
Remember the measuring wheel that we talked about at the start of this section? The tool works because each time the wheel rotates one time around, it covers a distance equal to its circumference. If the wheel is larger, then it will cover more distance with one rotation.
|
The circumference of a circle is distance around the circle. It is directly proportional to the length of the radius of the circle. The circumference is equal to 2π times the radius, or π times the diameter.
|
(Note: the value of π is approximately 3.14. You should, however, use the π key on your calculator when evaluating expressions. Your answers will be more accurate.)
Example:
What is the circumference of a circle with radius 35 cm?
Solution:
C = 2πr = 2π(35) = 70π ≈ 219.91 cm
Example:
What is the radius of a circle if the circumference is 69.12 in.?
Solution:
C = 2πr
69.12 = 2πr
*Using your calculator, enter 69.12, then divide by (2π) using the parentheses.
11 = r
The radius is 11 in.
Example:
Find the circumference of the following circle:
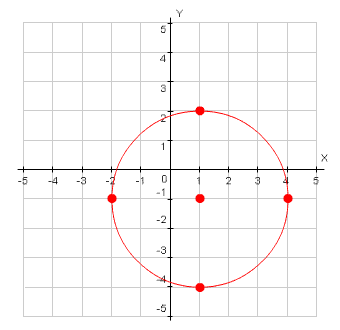
Graph of Circle
Solution:
From the graph, you can see that the radius is 3.
C = 2πr = 2π(3) = 6π ≈ 18.85 units