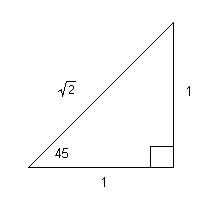
Using Special Right Triangles

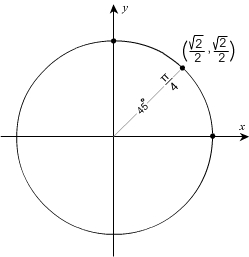
Let’s put all of the information we have leaned thus far to find the cosine and sine of 45°.

We can build the following right triangle:

P(cos 45°, sin 45°)

You have now found one point on the unit circle.