Quadrant I of the Unit Circle
The following is the first quadrant of the unit circle.

Remember that a unit circle has a radius of 1 unit. The angles in the first quadrant include 30° or 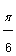 , 45° or
, 45° or 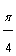 , and 60° or
, and 60° or 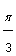 . How can we find cosine and sine of these angles using the unit circle?
. How can we find cosine and sine of these angles using the unit circle?
The answer is that we can build special right triangles. We know that any point P on a unit circle will have an x and a y coordinate. We also know that the x and y values in Quadrant I are both positive. In the case of the unit circle the following is true:
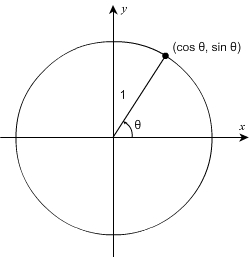
This means that P(x, y) is equivalent to P(cos θ, sin θ).x = cos θ
y = sin θ
and
x2 + y2 = r2 or x2 + y2 = 1 since the radius of a unit circle is 1. This equation comes from the Pythagorean Theorem.