Triangle Rules
Sum of Angles
The sum of the angles in any plane triangle is 180°.
Similar Triangles
Two triangles are said to be similar if all three of their angles are equal. Similar triangles thus have the same basic shape but may be different sizes and have different orientations. Two useful theorems about similar triangles are:
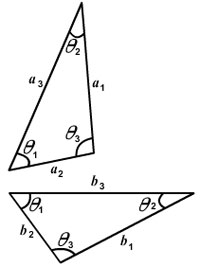
- Two triangles are similar if any two of the angles are equal. (This follows because the third angles must also be equal since the sum of the angles of a triangle is 180°.) That is,
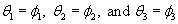
- The ratios of corresponding sides of two similar triangles are equal. That is,
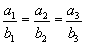
Congruent Triangles
Two triangles are congruent if one can be placed precisely on top of the other. That is, they are similar triangles and they have the same size. Two triangles are congruent if any of the following holds:
- The three corresponding sides are equal (side-side-side).
- Two sides and the enclosed angle are equal (side-angle-side).
- Two angles and the enclosed side are equal (angle-side-angle).