Equations of a Circle Centered at the Origin
In this section, we are going to study how to write the equation that defines a circle in the coordinate plane. We are also going to study how to graph a circle if we are given information about the circle, such as the center and the radius.
To begin, let’s look at a very basic circle, one that has its center at the origin.
Circle centered at the origin with radius r
Notice that every point along the circle is a distance ‘r’ away from the center. This is the radius.
Each point on the circle can also be defined by x- and y-coordinates. The relationship between the x- and y-coordinates and the radius can be found using a right triangle.
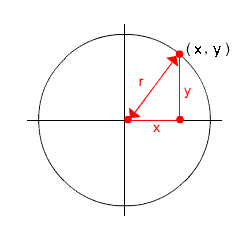
Circle centered at the origin
Starting at the origin, the x-value is the horizontal distance and the y-value is the vertical distance. These, along with the radius, make a right triangle. Using the Pythagorean Theorem, we can write the equation:
|
x2 + y2 = r2 This is the general equation of a circle that is centered at the origin. |
Example:
What is the equation of the circle centered at the origin with radius 4?
Solution:
We are told that r = 4.
The general equation is x2 + y2 = r2, so the equation when r = 4 is:
x2 + y2 = 42
x2 + y2 = 16
Example:
What is the equation of the circle centered at the origin with diameter 10?
Solution:
We are told that d = 10. Since the radius is one-half of the diameter, r = 5.
The general equation is x2 + y2 = r2, so the equation when r = 5 is:
x2 + y2 = 52
x2 + y2 = 25