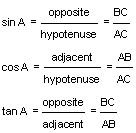Trigonometric Functions: Right Triangle Trigonometry

Trigonometric Ratios

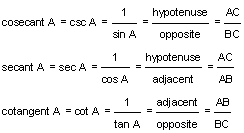
There are six trigonometric ratios that we need to discuss, but three are built directly off the other three. These ratios are sine, cosine, tangent, cosecant, secant, and cotangent.

Let’s look at our right triangle and define these trigonometric ratios in terms of angle A.

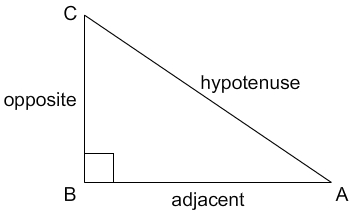
Right triangle with adjacent side, opposite side and hypotenuse from angle A.

Sine of A equals opposite divided by hypotenuse equals BC divided by AC. Cosine of A equals adjacent divided by hypotenuse equals AB divided by AC. Tangent of A equals opposite divided by adjacent equals BC divided by AB

The ratio sine can be written as sin, cosine is written as cos, and tangent is written as tan. An easy way to remember these three ratios is the use the acronym SOHCAHTOA.

S = Sine
O = Opposite
H = Hypotenuse
C = Cosine
A = Adjacent
H = Hypotenuse
T = Tangent
O = Opposite
A = Adjacent

The trigonometric ratios cosecant, secant, and cotangent are reciprocals of the sine, cosine, and tangent functions, respectively.

Cosecant of A, written csc A, equals 1 divided by sine of A equals hypotenuse divided by opposite equals AC divided by BC. Secant of A, written sec A, equals 1 divided by cosine of A equals hypotenuse divided by adjacent equals AC divided by AB. Cotangent of A, written cot A, equals 1 divided by tangent of A equals adjacent divided by opposite equals AB divided by BC